Crystal field theory

Crystal field theory (CFT) is a model that describes the breaking of degeneracies of electronic orbital states, usually d or f orbitals, due to a static electric field produced by a surrounding charge distribution (anion neighbors). This theory has been used to describe various spectroscopies of transition metal coordination complexes, in particular optical spectra (colours). CFT successfully accounts for some magnetic properties, colours, hydration enthalpies, and spinel structures of transition metal complexes, but it does not attempt to describe bonding. CFT was developed by physicists Hans Bethe and John Hasbrouck van Vleck in the 1930s. CFT was subsequently combined with molecular orbital theory to form the more realistic and complex ligand field theory (LFT), which delivers insight into the process of chemical bonding in transition metal complexes.
At almost exactly the same time that chemists were developing the valence-bond model for coordination complexes, physicists such as Hans Bethe, John Van Vleck, and Leslie Orgel were developing an alternative known as crystal field theory. This theory tried to describe the effect of the electrical field of neighboring ions on the energies of the valence orbitals of an ion in a crystal. Crystal field theory was developed by considering two compounds: manganese(II) oxide, MnO, and copper(I) chloride, CuCl.

Octahedral Crystal Field

Each Mn2+ ion in manganese(II) oxide is surrounded by six O2- ions arranged toward the corners of an octahedron, as shown in the figure below. MnO is therefore a model for an octahedral complex in which a transition-metal ion is coordinated to six ligands.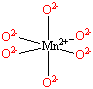


What happens to the energies of the 4s and 4p orbitals on an Mn2+ ion when this ion is buried in an MnO crystal? Repulsion between electrons that might be added to these orbitals and the electrons on the six O2- ions that surround the metal ion in MnO increase the energies of these orbitals. The three 4p orbitals are still degenerate, however. These orbitals still have the same energy because each 4p orbital points toward two O2- ions at the corners of the octahedron.